|
Indefinite Delivery-indefinite Quantity, Indefinite Quantity, Indefinite Delivery Contract
{{disambig ...
Indefinite may refer to: * the opposite of definite in grammar ** indefinite article ** indefinite pronoun * Indefinite integral, another name for the antiderivative * Indefinite forms in algebra, see definite quadratic forms * an indefinite matrix See also * Eternity * NaN * Undefined (other) Undefined may refer to: Mathematics * Undefined (mathematics), with several related meanings ** Indeterminate form, in calculus Computing * Undefined behavior, computer code whose behavior is not specified under certain conditions * Undefin ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Definiteness
In linguistics, definiteness is a semantic feature of noun phrases, distinguishing between referents or senses that are identifiable in a given context (definite noun phrases) and those which are not (indefinite noun phrases). The prototypical definite noun phrase picks out a unique, familiar, specific referent such as ''the sun'' or ''Australia'', as opposed to indefinite examples like ''an idea'' or ''some fish''. There is considerable variation in the expression of definiteness across languages, and some languages such as Japanese do not generally mark it so that the same expression could be definite in some contexts and indefinite in others. In other languages, such as English, it is usually marked by the selection of determiner (e.g., ''the'' vs ''a''). In still other languages, such as Danish, definiteness is marked morphologically. Definiteness as a grammatical category There are times when a grammatically marked definite NP is not in fact identifiable. For example, ' ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Indefinite Article
An article is any member of a class of dedicated words that are used with noun phrases to mark the identifiability of the referents of the noun phrases. The category of articles constitutes a part of speech. In English, both "the" and "a(n)" are articles, which combine with nouns to form noun phrases. Articles typically specify the grammatical definiteness of the noun phrase, but in many languages, they carry additional grammatical information such as gender, number, and case. Articles are part of a broader category called determiners, which also include demonstratives, possessive determiners, and quantifiers. In linguistic interlinear glossing, articles are abbreviated as . Types Definite article A definite article is an article that marks a definite noun phrase. Definite articles such as English ''the'' are used to refer to a particular member of a group. It may be something that the speaker has already mentioned or it may be otherwise something uniquely specified. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Indefinite Pronoun
An indefinite pronoun is a pronoun which does not have a specific familiar referent. Indefinite pronouns are in contrast to definite pronouns. Indefinite pronouns can represent either count nouns or noncount nouns. They often have related forms across these categories: universal (such as ''everyone'', ''everything''), assertive existential (such as ''somebody'', ''something''), elective existential (such as ''anyone'', ''anything''), and negative (such as ''nobody'', ''nothing''). Many languages distinguish forms of indefinites used in affirmative contexts from those used in non-affirmative contexts. For instance, English "something" can be used only in affirmative contexts while "anything" is used otherwise. Indefinite pronouns are associated with indefinite determiners of a similar or identical form (such as ''every'', ''any'', ''all'', ''some''). A pronoun can be thought of as ''replacing'' a noun phrase, while a determiner ''introduces'' a noun phrase and precedes any a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
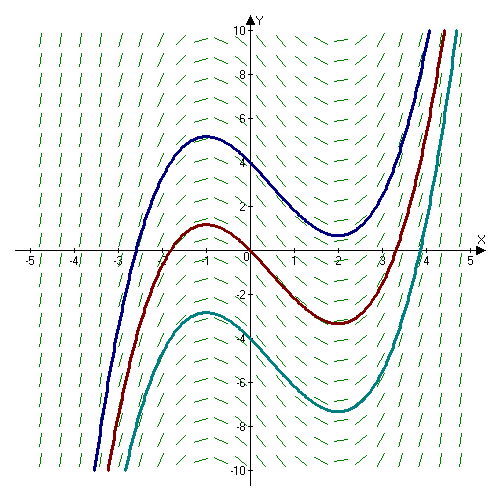
Antiderivative
In calculus, an antiderivative, inverse derivative, primitive function, primitive integral or indefinite integral of a function is a differentiable function whose derivative is equal to the original function . This can be stated symbolically as . The process of solving for antiderivatives is called antidifferentiation (or indefinite integration), and its opposite operation is called ''differentiation'', which is the process of finding a derivative. Antiderivatives are often denoted by capital Roman letters such as and . Antiderivatives are related to definite integrals through the second fundamental theorem of calculus: the definite integral of a function over a closed interval In mathematics, a (real) interval is a set of real numbers that contains all real numbers lying between any two numbers of the set. For example, the set of numbers satisfying is an interval which contains , , and all numbers in between. Other ... where the function is Riemann integrable is eq ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Definite Quadratic Form
In linguistics, definiteness is a semantic feature of noun phrases, distinguishing between referents or senses that are identifiable in a given context (definite noun phrases) and those which are not (indefinite noun phrases). The prototypical definite noun phrase picks out a unique, familiar, specific referent such as ''the sun'' or ''Australia'', as opposed to indefinite examples like ''an idea'' or ''some fish''. There is considerable variation in the expression of definiteness across languages, and some languages such as Japanese do not generally mark it so that the same expression could be definite in some contexts and indefinite in others. In other languages, such as English, it is usually marked by the selection of determiner (e.g., ''the'' vs ''a''). In still other languages, such as Danish, definiteness is marked morphologically. Definiteness as a grammatical category There are times when a grammatically marked definite NP is not in fact identifiable. For example, ' ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Indefinite Matrix
In mathematics, a symmetric matrix M with real entries is positive-definite if the real number z^\textsfMz is positive for every nonzero real column vector z, where z^\textsf is the transpose of More generally, a Hermitian matrix (that is, a complex matrix equal to its conjugate transpose) is positive-definite if the real number z^* Mz is positive for every nonzero complex column vector z, where z^* denotes the conjugate transpose of z. Positive semi-definite matrices are defined similarly, except that the scalars z^\textsfMz and z^* Mz are required to be positive ''or zero'' (that is, nonnegative). Negative-definite and negative semi-definite matrices are defined analogously. A matrix that is not positive semi-definite and not negative semi-definite is sometimes called indefinite. A matrix is thus positive-definite if and only if it is the matrix of a positive-definite quadratic form or Hermitian form. In other words, a matrix is positive-definite if and only if it defines a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Eternity
Eternity, in common parlance, means Infinity, infinite time that never ends or the quality, condition, or fact of being everlasting or eternal. Classical philosophy, however, defines eternity as what is timeless or exists outside time, whereas sempiternity corresponds to infinite Duration (philosophy), duration. Philosophy Classical philosophy defines eternity as what exists outside time, as in describing timeless supernatural beings and forces, distinguished from sempiternity which corresponds to infinite time, as described in requiem prayers for the dead. Some thinkers, such as Aristotle, suggest the Eternity of the world, eternity of the natural cosmos in regard to both past and future eternal duration. Boethius defined eternity as "simultaneously full and perfect possession of interminable life". Thomas Aquinas believed that God's eternity does not cease, as it is without either a beginning or an end; the concept of eternity is of divine simplicity, thus incapable of be ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |